Work

In physics, work is defined as the product of the force applied to an object and the object’s displacement in the direction of the force. In other words, work is the transfer of energy from or to an object when a force acts on it and causes the thing to move.

The formal mathematical definition of work is:

Where W is the work done, F is the magnitude of the force, d is the object’s displacement, and theta is the angle between the force’s direction and the displacement’s direction.

Work is a scalar quantity, which means it has only magnitude and no direction. It is typically measured in joules (J) or newton-meters (N-m). The concept of work is essential in physics and engineering, as it is related to energy and the ability to do work or transfer energy from one system to another.

In simpler terms.

Work measures how much energy is required to move an object by applying force. When a force is applied to an object and moves in the direction of the force, work is said to be done on the object. The amount of work done is equal to the force applied multiplied by the distance the object moved in the direction of the force.

For example, if you lift a 10 kg object at a distance of 1 meter against the force of gravity, you are doing work on the object. The amount of work you do is equal to the force you are applying (similar to the weight of the object, which is approximately 98 Newtons) multiplied by the distance you are lifting it (1 meter). So, the work done on the object would be 98 Joules (J).

In simpler terms, work is a measure of the effort required to move an object, and it is calculated as the product of the force applied, and the distance moved in the direction of the force.

Work done by a constant force

When a constant force is applied to an object and moves a certain distance in the direction of the force, the work done on the object can be calculated using a simple formula.

The work done by a constant force can be calculated as follows:

where W is the work done, F is the magnitude of the force, d is the distance moved in the direction of the force, and theta is the angle between the force vector and the displacement vector.

If the force is applied in the same direction as the displacement, the angle between them is zero, and cos(theta) = 1, so the formula reduces to:

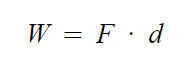
In other words, the work done by a constant force is equal to the magnitude of the force multiplied by the distance moved in the direction of the force.

For example, if a force of 50 Newtons is applied to an object, and it moves a distance of 2 meters in the direction of the force, the work done on the object is:

W = F * d W = 50 N * 2 m W = 100 J

So the work done on the object is 100 Joules.

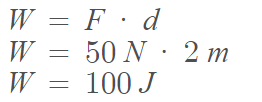
Problems:

1. A force of 20 Newtons is applied to an object, and it moves a distance of 5 meters in the direction of the force. What is the work done on the object?
2. A car engine exerts a force of 5000 N on the car, and the car moves a distance of 200 meters in the direction of the force. What is the work done by the engine on the car?
3. A weightlifter lifts a barbell weighing 200 kg a distance of 1 meter, using a force of 500 Newtons. What is the work done by the weightlifter on the barbell?
4. A crate is pulled along a horizontal surface by a force of 100 N, and it moves a distance of 10 meters in the direction of the force. What is the work done on the crate?
5. A rock climber pulls herself up a cliff by applying a force of 500 N to the rope, and she ascends a distance of 20 meters. What is the work done by the climber on the rope?

[read more]

1. W = F * d = 20 N * 5 m = 100 J. The work done on the object is 100 Joules.
2. W = F * d = 5000 N * 200 m = 1,000,000 J. The work done by the engine on the car is 1,000,000 Joules.
3. W = F * d = 500 N * 1 m = 500 J. The work done by the weightlifter on the barbell is 500 Joules.
4. W = F * d = 100 N * 10 m = 1000 J. The work done on the crate is 1000 Joules.
5. W = F * d = 500 N * 20 m = 10,000 J. The work done by the climber on the rope is 10,000 Joule.s

[/read]

Exercises:

1. A crate is pushed a distance of 4 meters along a rough surface by a force of 50 Newtons. If the frictional force opposing the motion is 20 Newtons, what is the work done on the crate?
2. A shopper pushes a cart with a force of 20 Newtons over a distance of 25 meters. If the angle between the direction of the force and the direction of the displacement is 30 degrees, what is the work done by the shopper?
3. A force of 50 Newtons compresses a spring with a spring constant of 100 N/m. If the spring is compressed a distance of 0.2 meters, what is the work done on the spring?
4. A student lifts a 10 kg weight from the floor to a height of 2 meters, using a force of 50 Newtons. What is the work done by the student on the weight? Research on formula of work against gravity.
5. A car is lifted a distance of 2 meters by a hydraulic lift, using a force of 5000 Newtons. What is the work done on the car by the hydraulic lift?

More problems

1. A 20 kg box is pulled along a rough surface by a force of 80 Newtons. If the coefficient of kinetic friction is 0.3, and the box moves a distance of 5 meters, what is the work done on the box?
2. A rocket is launched with an initial acceleration of 10 m/s^2. If the rocket has a mass of 1000 kg and the force of the rocket engine is constant at 100,000 Newtons, what is the work done by the engine after the rocket has accelerated to a speed of 500 m/s?
3. A person pushes a car up a hill with a force of 500 Newtons, moving it a distance of 50 meters along a slope that makes an angle of 30 degrees with the horizontal. If the car has a mass of 1000 kg and starts from rest, what is its final speed at the end of the 50-meter distance?
4. A 5 kg weight is lifted a distance of 10 meters, using a force of 200 Newtons. If the weight is lifted at a constant speed, what is the work done on the weight?
5. A block is pulled up a ramp that makes an angle of 45 degrees with the horizontal, using a force of 50 Newtons. The block has a mass of 10 kg, and the ramp is 6 meters long. If the block starts from rest at the bottom of the ramp, what is its speed at the top of the ramp? (Assume no frictional losses.)
   
   Notes:

1. W = F * d * cos(theta) for calculating the work done by a force at an angle to the displacement vector.
2. W = ΔK for calculating the work-energy theorem, where ΔK is an object’s kinetic energy change.
3. F = ma for calculating the force on an object based on its mass and acceleration, where a is the object’s acceleration.
4. KE = 1/2 mv^2 for calculating the kinetic energy of an object, where m is the mass of the object and v is its velocity.
   

[read more]

1. The work done on the box is equal to the force applied minus the work done by friction. The force applied is 80 N, and the work done by friction is (0.3)(20 kg)(9.8 m/s^2)(5 m) = 294 J. So, the work done on the box is 80 N * 5 m – 294 J = 406 J.
2. The work done by the engine is equal to the change in kinetic energy of the rocket. The initial kinetic energy of the rocket is zero, and the final kinetic energy of the rocket is (1/2)(1000 kg)(500 m/s)^2 = 62.5 million J. So, the work done by the engine is 62.5 million J.
3. The force applied on the car is F = ma, where a is the acceleration of the car up the slope. The component of the force along the slope is F * sin(30) = 250 N. The work done by the force is (250 N)(50 m) = 12,500 J. The final kinetic energy of the car is equal to the work done by the force, so (1/2)(1000 kg)(v^2) = 12,500 J. Solving for v, we get v = 5 m/s.
4. Since the weight is lifted at a constant speed, the work done on the weight is equal to the change in gravitational potential energy, which is mgh, where m is the mass of the weight, g is the acceleration due to gravity, and h is the height lifted. The work done on the weight is (5 kg)(9.8 m/s^2)(10 m) = 490 J.
5. The work done by the force is equal to the change in kinetic energy of the block. The work done by the force is (50 N)(6 m) = 300 J. The change in potential energy of the block is mgh, where h is the height of the ramp. Since the ramp makes an angle of 45 degrees with the horizontal, h = (6 m)(sin 45) = 4.24 m. The change in potential energy is (10 kg)(9.8 m/s^2)(4.24 m) = 416 J. The total work done on the block is the sum of the work done by the force and the change in potential energy, or 716 J. The final kinetic energy of the block is equal to the work done, so (1/2)(10 kg)(v^2) = 716 J. Solving for v, we get v = 11.9 m/s (to two significant figures).

[/read]

More exercises:

1. A 10 kg block is pushed along a surface with a force of 50 N at an angle of 30 degrees to the horizontal. The coefficient of kinetic friction between the block and the surface is 0.2, and the block moves a distance of 4 meters. What is the work done on the block by the force?
2. A car is pulled up a slope at a constant speed with a force of 500 N. If the slope makes an angle of 20 degrees with the horizontal and the car moves a distance of 100 meters, what is the work done on the car by the force?
3. A force of 20 N is applied to a 2 kg object at an angle of 60 degrees to the horizontal, and the object moves a distance of 3 meters in the direction of the force. What is the work done on the object by the force?
4. A 5 kg weight is lifted a distance of 2 meters, using a force of 50 N. If the weight is lifted at a constant speed, what is the work done on the weight?
5. A block is pushed up a ramp that makes an angle of 30 degrees with the horizontal, using a force of 20 N. The block has a mass of 2 kg, and the ramp is 4 meters long. If the block starts from rest at the bottom of the ramp, what is its speed at the top of the ramp? (Assume no frictional losses.)

References:

1. Sears, F., & Zemansky, M. (2016). University physics. Pearson.
2. Halliday, D., Resnick, R., & Walker, J. (2013). Fundamentals of physics. John Wiley & Sons.
3. Nave, C. R. (2021). HyperPhysics. Georgia State University. http://hyperphysics.phy-astr.gsu.edu/hbase/hph.html
4. The Physics Classroom. (n.d.). http://www.physicsclassroom.com/
5. Khan Academy. (n.d.). Work and energy. https://www.khanacademy.org/science/physics/work-and-energy